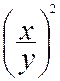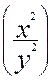DOC. INFO : Professeur - Formateur

25 / 26

DOC : livre  Elève .Cours  interactifs - et travaux +  corrigés.

TITRE :   «  Carrés » d ' opérations simples .Leçon  4/5  .

DOSSIER  N°25 COURS  INTERACTIF «  Carrés » d ' opérations simples .Leçon  4/5  .

Information « TRAVAUX » Cliquer sur  le mot !.

INFORMATIONS PEDAGOGIQUES :

NIVEAU : Formation  Niveau V  (inclus le CAP et CFA) Objectif :  n° 4/5 sur les carrés	OBJECTIFS : =savoir  calculer les carrés d’opérations simples. Prépare les Identités Remarquables , utilisées pour résoudre les équations du second degré .

I ) Pré requis:

 

puissance d' un nombre

II ) ENVIRONNEMENT du dossier :

Index  warmaths	Objectif précédent : 1°)   2°) le « carré » d’un nombre. 3°) Les I R  déjà vu : cours N°7	Objectif suivant : 1°) Encadrement 2°) généralités sur les Puissances d’opérations simples 3°) les racines carrées d’opérations simples Vu : les Identités remarquables ( carrés d’une somme ; carrée d’une différence) voir : les IR et le calcul mental	1°) Tableau           75a 2°) tableau 3°) liste des cours.   voir : l’exposant 0
		Sciences

III )  LECON  n° 25  «  Carrés » d ' opérations simples .

CHAPITRES :

I ) CARRE d’une somme :  ( voir : calcul mental )	
II ) CARRE d’une différence : (voir : calcul mental )	
III )  CARRE d’un  produit  : ( a  b )2	
IV )  CARRE  d’un quotient ; d’une division ( fraction)  : ( a : b )2  ou ( a / b )2  ou ()2	
V  ) puissance au carré	

IV)   INFORMATIONS  «  formation leçon » :

Test	COURS	Travaux  auto - formation.			Corrigé des travaux  auto - formation.
		Contrôle	évaluation	Interdisciplinarité.	Corrigé Contrôle	Corrigé évaluation

 

 

Info plus  sur les puissances et racines Nièmes

 

V )   DEVOIRS  ( écrits):

Devoir diagnostique L tests.	
Devoir  Auto  - formatif  ( intégré au cours)	
Devoir Formatif  « Contrôle : savoir » ;   ( remédiation)	
Devoir  Formatif  «  Evaluation   savoir faire »  ( remédiation)	
Devoir sommatif .	
Devoir certificatif : ( remédiation )	

* remédiation : ces documents peuvent être réutilisés ( tout ou partie) pour conclure une formation .

 

Leçon	Titre
N°25	LES  PUISSANCES  « CARREES » d ' opérations simples

CHAPITRES

I ) CARRE d’une somme :  voir : calcul mental	
II ) CARRE d’une différence : voir : calcul mental	
III )  CARRE d’un  produit  : ( a  b )2	
IV )  CARRE  d’un quotient ; d’une division ( fraction)  : ( a : b )2  ou ( a / b )2  ou ()2	
V  ) puissance au carré	

 

COURS

 

Nous prenons le cas où les  deux nombres différents « a » et « b » :

 

I ) CARRE d’une somme :  voir : calcul mental



 

( a + b ) (a + b)   qui s’écrit   aussi  ( a + b )2 :le développement de  ( a + b )2   est égal à    a2  + 2ab + b2

SOS COURS

 

Application numérique :
Si (a ;b)	(a + b )2	a2  + 2ab + b2		conclusion
( 2 ;3)	(2 + 3 )2 = 25	22  + 223 + 32= 4 + 12 + 9 =  25		(a + b )2 = a2 + 2ab + b2
Forme algébrique :
( x ; y )	(x + y )2	x2  + 2xy + y2	(x + y )2 = x2+ 2xy + y2

 

Autres applications :

Application numérique

a)  Calcul de ( 30 + 2 )²

On pose :   ( 30 + 2 )²   = 30²  + 2 fois 30 fois 2  + 2²

calculs intermédiaires :

30²  = 900 ; 2 fois 30 fois 2 = 120 ; 2²   = 4

( 30 + 2 )²   = 900  +  120  + 4

calculs intermédiaires :

900 + 120 + 4  = 1024

( 30 + 2 )²   = 1024

Application algébrique :

b)   ( x +1 ) ( x + 1 )  qui s’écrit        ( x + 1 ) ² = x²  + 2x + 4

 

c)   ( 3x + 2 ) ( 3x + 2 ) qui s’écrit   ( 3x + 2 ) ²  =  9x²  + 12x + 4

 

II ) CARRE d’une différence : voir : calcul mental



 

 

( a - b ) ( a - b ) qui s’écrit   aussi   ( a - b) 2   :  le développement de ( a - b) 2  est égal à   a2 –  2ab +  b2

SOS COURS

 

Application numérique :
Si (a ;b)	(a - b )2	a2  - 2ab + b2	conclusion
( 5 ;2)	(5 –2  )2 = 32 = 9	52  -  252 + 22= 25 – 20  + 4 =  9	(a - b )2 = a2 - 2ab + b2
Forme algébrique :
( x ;y)	(x - y )2	x2  - 2xy + y2	(x + y )2 = x2- 2xy + y2

 

A )  Application calcul : ( 30 - 1 )²

 

On pose :   ( 30 - 1 )²   = 30²  - 2 fois 30 fois 1  + 1²

calculs intermédiaires :

30²  = 900 ; 2 fois 30 fois 1 = 60 ; 1²   =  1

( 30 + 2 )²   = 900  - 60  + 1

calculs intermédiaires :

900 - 60 + 1  = 940 + 1  = 941

( 30 -  2 )²   = 941

 

B ) Application « algèbre »

Exemples :  

a)  ( x -1 ) ( x - 1 )  qui s’écrit   ( x - 1 ) ² = x² –2x + 1

 b)  ( 3x - 2 ) ( 3x - 2 ) qui s’écrit   ( 3x - 2 ) ²   =  9 x²  - 12x + 4

 

 

III )  CARRE d’un  produit  : ( a  b )2



 

A retenir :  ( a  b )²     =   a ² b²

 

a ; b	( a  b )2	a 2 b2	conclusion
2 ; 3	( 2  3)2   = ( 6 ) 2 = 36	2 2 32  = 4 9  = 36	( a  b )2  = a 2 b2
2,5 ; 3,2	( 2,5  3,2 )2 =	2,5 2 3,22 =
x ; y	( x  y )2  =  (xy)2	x 2 y2	( x  y )2   =   x 2 y2

 

Applications numériques :

( 5  10 ) ²  =

( 5  10² ) ²  =

( 3  10 ⁴) ²  =

 

 

 

 

 

IV )  CARRE  d’un quotient ; d’une division ( fraction) : ( a : b )2  ou (  a / b )2  ou ()2



Exemples types :

Soit  « a » et « b »	=	=	Conclusion :
3 ; 2	()2  = ( 1,5 ) 2 = 2,25	=   = 2,25	=
2,5 ; 3,2	A vous de faire ! !
x ; y			=

Applications  Numériques :

 

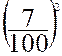 =     = 0,49

 

 

Remarques :

Ecriture de puissances : puissances négatives

1°)  peut s’écrire   b^-2     ;  

exemples :  peut s’écrire   2^-2  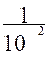 ; peut s’écrire   10^-2

2° )  peut s’écrire  = a b^-2 ;  peut s’écrire  = 2 10^-2 ; par extension   peut s’écrire  = 8 10 ^-2 

3°)    peut s’écrire   : a² b^-2 ;   peut s’écrire   : 2² 10^-2

Cas particuliers :

A ) 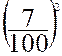   = =    = 0,49   ou  4910^-2

 

 

 

 

 

 

 

 

 

V )  CARRE d’une puissance : ( a n )2

 

« a » et « n »	( a n )2	a 2n	Conclusion :
2 ; 3	( 2 3 )2  = 82 ; (= 64)	2 32  =2 6  =   64	( a n )2= a 2n
2,5 ; 3	( 2,5 3  )2  = 244,14062
x ; y	( x y )2  =  x 2y

 

a )   (10^-3 ) ²  =   10^-6

b)    (10^{-2 ) 2}  =   10^-4

c)      ( 8¹) ²  =  ( 8²  )

d)      ( 2³) ²  =   ( 2⁶ ) 

Pour les exercices suivants voir la puissance d’une puissance

e )   ( 8  10^-3 ) ²  =  ( 8²  10^-6 )   =  64 10^-6

f )  ( 2³  10^-2 ) ²  =   ( 2⁶  10^-4 )  =   64 10^-4

 

 

Leçon	TRAVAUX d ’ AUTO - FORMATION sur
N°25	LES  PUISSANCES  « CARREES » d ' opérations simples

 

TRAVAUX  N° 25   d ’ AUTO - FORMATION : CONTROLE:

 

I°) Transformer les égalités suivantes : 

( a + b )²  =
( a – b  )2  =
( a2 )2  =
( an )2  =
=
=
=
b-2 =
a b-2     =
a2 b-2    =
=

 

TRAVAUX N°25    d ‘ AUTO - FORMATION    EVALUATION :

 

1°)  en utilisant les relations des  IR  , transformer et calculer :

( 30 + 2 )²  =  

( 90 + 3 )²  =  

( 30 – 1 )²  =  

( 90 –3 )²  =  

 

2°) en utilisant  la relation    (aⁿ)² ; transformer et calculer :

( 10² )²  =  

( 10³ )²  =  

 

 3°) en vous aidant des  relations suivantes

 =          ;    =   b^-2 ;            = a b^-2       et     = a² b^-2 ;

4°)  transformer et calculer :

   =

  =

   =  

  =  

 

 

INTERDISCIPLINARITE

 

 

 

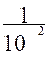VOIR FICHE UTILISATION DE LA CALCULATRICE