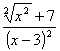LES FONCTIONS ( INFORMATIONS)

Prérequis:

Voir : calcul de la valeur numérique d’une expression algébrique.

 

Les nombres relatifs

CALCUL NUMERIQUE

ENVIRONNEMENT du dossier:

INDEX    

Objectif précédent  

Objectif suivant

Tableau        4.01

DOSSIER:   Calculs :recherche de valeurs

 

 

 

 

CORRIGE

 

A )On donne  l’équation  y = 3,5 x  ; calculer :

si x = 2

alors y = 7

si x =  -2

alors y =  -7

si x = 3/7

alors y =10,5 /7 ; = 1,5

si x = 5

alors y = 17,5

si x =  - 3/4

alors y = 2,625

Si x = 2,75
Alors y = 9,625

 

B) On donne  l’équation de la forme :  y = a x ; calculer :

si x = 4

et  y = 67 +15 – 20 =

alors     a = 1,5

si x =-2,7

et  y = 3,2

alors      a = - 3,2 /2,7 ;

-1,1851852

 

transformer l ’ égalité :

si   y = a x     ;   alors a =            (on dit :exprimer « a » en fonction de « y » et « x » ; ou autrement dit :  exprimer « a » avec « y » et « x »   ) 

 

 

C)   trouver la valeur de « y » si l’on donne une valeur  à «a ; x ; b » dans les cas suivants :

(remplir le tableau suivant)Forme  y = ax +b

 

a =

x =

b  =

y = ax + b

Résultat  y =

3

+2

+2

3 2+2

8

- 3

+2

+2

-32+2

-4

0,5

-2

+2

0,5-2+2

1

-1,5

-2

+3

-1,5-2+3

6

1 / 3

1

-0,5

1/31-0,5

-0,166666666

- 2 / 3

3

1,5

-(2/3) 3+1,5

-0-5

 

D )Savoir résoudre les équations du premier :

SOS COURS

45 = 0,5 x

x   =  90

18 =  

 

x   =   36

16= x + 0,5          

 

x   =  15,5

- 4,6 = 2,5 x + 1,3

 

x   =  -2,36

2,4 = +1,8

 

x   =  -1,8

1,6 = -2 ,9 x

 

x   =  - 0,551

Résoudre une équation du second degré à une inconnue

 

E )  CALCUL

Soit l'équation:

Poser l’opération

Calculer « y »

 

 

équation

Si x = 2

Alors y =

Y = 3x

 

6

Y =

 

-4/7

Y = 3 x + 5

 

11

Y =+2  ;

 

-10/7

Y =3 x2

 

12

Y =

 

1

Y =3 x2 + 5 x

 

22

Y =+7x

 

15

Y =3x2 +5x +1

 

26

Y =+7x+2

 

17

Y =

 

1,414

Y =

 

8,414

 

Partie 2 

 

I )  On considère  les équations   y1 = x2    ;  y2 = 2x2  ,  y3  = -x2 et  y4 = - 0,5x2.

Compléter le tableau

x

0

0,5

1

1,5

2

2,5

3

3,5

4

y1

0

0,25

1

2,25

4

6,25

9

12,25

16

y2

0

0,5

2

5,5

8

12,5

18

24,5

32

y3

0

-0,25

-1

-2,25

-4

-6,25

-9

-12,25

-16

y4

0

-0,125

-0,5

1,125

-2

-3,125

-4,5

-6,125

-8

 

II )  On considère les fonctions    f1 = y1 ;  f2= y2 ;    f3= y3   et y4 = f4, , telles que f1(x) = x2    f2(x) = 2x2  , f3(x) = -x2 et f 4(x) = - 0,5x2.

Compléter le tableau suivant:

 

x

0

0,2

0,5

0,8

1

2

3

4

5

f1(x)

0

0,04

0,25

0,64

1

4

9

16

25

f2(x)

0

0,08

0,5

1,28

2

8

18

32

50

f3(x)

0

-0,04

-0,25

-0,64

-1

-4

-9

-16

-25

f 4(x)

0

-0,02

-0,125

-0,32

-0,5

-2

-4,5

-8

-12,5

 

 

III )   On considère les fonctions f1 = y1 ; f2= y2 ;  f3= y3    et y4 = f4, , telles que f1(x) = x2    f2(x) = 2x2  , f3(x) = -x2 et f 4(x) = - 0,5x2.

Compléter le tableau suivant:

x

0

-0,2

-0,5

-0,8

-1

-2

-3

-4

-5

f1(x)

0

0,04

0,25

0,64

1

4

9

16

25

f2(x)

0

0,08

0,5

1,28

2

8

18

32

50

f3(x)

0

-0,04

-0,25

-0,64

-1

-4

-9

-16

-25

f 4(x)

0

-0,02

-0,125

-0,32

-0,5

-2

-4,5

-8

-12,5

 

Comparer avec le tableau précédent et conclure.